The NASA drawing shown above is an artist's depiction of the Space Shuttle Endeavour as it begins to deploy its 200-ft. (60 meter) mast in Earth orbit in preparation for radar mapping of Earth's topography. Heat shields for space shuttles, such as the Endeavour, are coated with a special protective covering to allow them to withstand the intense heat of blast off and re-entry. One such protective covering developed by NASA is called PCCM (Protective Coating for Ceramic Materials) and was developed at NASA Ames Research Center. NASA's primary application of this protective coating is for ceramic materials used in heat shields for space vehicles. The coating lowers the surface temperature of the thermal control structure and reduces heat transfer through the surface preventing degradation of the underlying ceramic material.
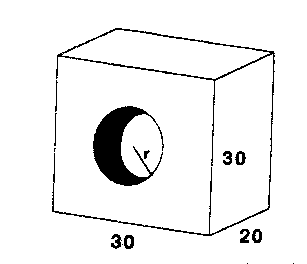
Activity: to determine surface area of a three dimensional object with changing dimensions. Mathematical Skills: grade level 9-12, work in three dimensions, surface area, development of algebraic equations, graphing parabolas, interpretation of data, and volume. Materials: Graph paper. A graphing utility is optional. While a manipulative is not required for this activity, a simple representation of the object can be constructed from wood. Directions: You are a research scientist working for the NASA development team with the PCCM. You are experimenting with PCCM to determine if it could be used in other industrial applications.
|