|
The graphing calculator can be used in
several ways to check your work
when you are dealing with inverse functions.
| 1. |
| Use the
calculator to quickly determine if the inverse of your graph
is a function. |
Graph the original function and observe the results of the horizontal line test.
Example: Is the inverse
of 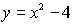 a function? a function?
Since this parabola does not pass the horizontal line
test
, the inverse will not be a function. |
The red lines will not appear on the
calculator.
|
|
| 2. |
Use the
calculator to determine if your algebraic inverse answer is
correct.
Method 1: |
|
| |
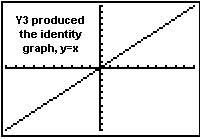
A quick way
to determine if your algebraic inverse answer is correct is to:
a.) enter your starting function in Y1.
b.) enter your algebraic inverse answer in
Y2.
c.) enter Y1(Y2) in
Y3. (composition of functions)
d.) GRAPH
e.) if Y3 produces the graph of
the identity function, y = x, your algebraic inverse answer is
correct.
 |
 |
| |
Use the
calculator to determine if your algebraic inverse answer is
correct.
Method 2: |
|
| |
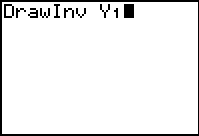
The graphing
calculator has a DrawInv command that will DRAW a function's inverse.
To use this feature, place the original starting function into
Y1. Press
2ndPRGM (DRAW) #8 DrawInv. The command will appear on the home screen
waiting for a parameter. Enter the location of the function to be
investigated, Y1. The graph of the inverse will appear. |
 |
| |
If you wish to use this feature to check an algebraic
answer,
a. Enter the original function into
Y1.
b. DrawInv Y1 (must be done before entering your algebraic answer)
c. Solve for the inverse algebraically on a piece of
paper.
|
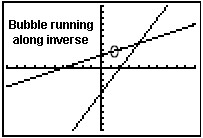
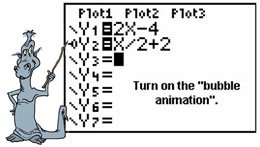
d. Enter your algebraic
answer for the inverse into Y2 with the bubble animation
(in front of
Y2), and hit GRAPH.
e. If your algebraic
answer is correct, the bubble animation will follow the
inverse graph drawn by the calculator.
 |
 |
|
|